Back to Daniel's Honor Project
'On Pi is wrong, and the Tauist Movement'
$ \pi $ is perhaps the most well known mathematical constant in the world, and its significance in mathematics cannot be overstated. $ \pi $ has been thoroughly studied by the most brilliant minds in the history of mathematics, is part of the most elementary mathematics education for the young students, and now is under attack by growing number of scientists who think that this magnificent constant is wrong.
Well, not really- $ \pi $ is still a transcendental number with decimal value 3.14159…. What is wrong is its undeserved adulation and sanctity. The constant deserving the glory, according to Bob Palais, professor at University of Utah and the author of the much discussed paper “Pi is wrong”, is $ \tau $.
Tal: Why $ \pi $? Geometrically, $ \pi $ is ratio of circle’s circumference to its diameter. This is unnatural – it is with radius, not diameter, that we define circles with. Therefore, I think it is more natural to describe the ration of circumference to the radius of the circle, $ circumference/radius $ = $ 2\pi $ = $ \tau $. Then the equation for circumference is simpler, namely $ Circumference = r\tau $.
Ip: Your insight is shortsighted, young grasshopper. You simplify the formula for circumference by sacrificing the formula for the area. With $ \tau $, the area of the circle now becomes A = 1/2 $ \tau r^2 $. What is so unnatural about diameter? Is the ratio of circumference, one full rotation around the circle, to radius, HALF across the circle, any more natural?
Tal: Perhaps not, but the area of the circle defined by $ \tau $, although a bit more bulky, serves an educational purpose. This comes in two fold: one is that it is now obvious that the area of the circle is the antiderivative of circumference, and the second is that the formula is not unfamiliar to us all. Where have you seen another formula bearing similar structure of $ 1/2xy^2 $? (think energy, gravity).
Ip: Well, that's a physicists' argument. They are strange.
Tal: That is beside the point. Another victory for $ \tau $ I can claim by the absurdity in defining one rotation of a circle as $ 2\pi $. How strange this idea is- half of rotation of circle is $ \pi $, quarter of a rotation is $ \pi /2 $. It is as if we define an hour of time with thirty minutes in our clocks. Half an hour is not quarter of a circle, quarter till the hour is now 1/8 of the circle before the next hour. This is truly an inconvenient notation.
Ip: But think not of angles but in areas. The most important circle of all, the unit circle, has an area of $ \pi $. Can you tell me the area of a quarter of a circle is? I say it is quarter of a $ \pi $. I suggest you don't waste your time since you think it is too much work to do simple multiplication with $ \pi $ in calculating the circumference..
Tal: Ip, you are acting like an ass today.
Ip: Sorry, I did not do well on trigonometry exam today and feel a bit irascible.
Tal: Well, you may not have if we had adopted $ \tau $! Periods of trig functions, sine and cosine, can be expressed as one tau!
Ip: Please- then the period of tangent and cotangent is $ \pi $. I would rather stick with a whole integer before I resort to those nasty fractions.
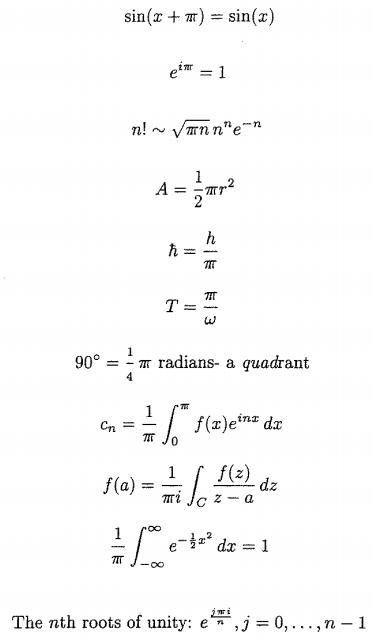
Tal: But you must deal with too many instances where you must deal with unnecessary 2! Professor Palais noted that "... Cauchy's integral formula and Fourier series formulas all begin with 1/2$ \pi $, Stirling's approximation and the Gaussian normal distribution both carry it, the Gauss-Bonnet and Picard theorems have the mark of $ 2\pi $... The blight of factors of 2 even affects physics, for example, in Maxwell's equation, Planck's constant. Here are few equations replacing $ \pi $ with $ \tau $
Ip: Okay.
Tal: So $ \tau $ instead of $ \pi $ it is?
Ip: No.
Tal: Why?
Ip: Because $ e^{\pi i} + 1 = 0 $ is still the most beautiful equation.
Tal: I think $ e^{\tau i} = 1 $ is more beautiful.
Ip: WHAT?
Tal: Yes. If you are fretting about the zero, then $ e^{\tau i} = 1 + 0 $
Ip: I don't think we can be friends anymore.
Finally, we compare the song of pi to song of tau